Copolymers
Overview
Radical polymerization is one of the most widely used polymerization methods in industry. This method relies on a chain-growth mechanism, in which monomers react consecutively by a free radical species. When radical polymerization is carried out with mixtures of two or more monomers, polymer products contain these monomers in the polymer chain form. Such a polymerization process is termed copolymerization, and the product is a copolymer. Copolymerization is widely used in industry because of its ability to tailor-make polymer products by imparting various functions, such as polarity, reacting groups, and toughness, to polymer materials derived from monomers. Since the structure of copolymers and, thus, the properties of the resulting polymer materials are determined by the reactivity of each monomer, understanding and predicting the monomer reactivity is an important issue in polymer science. Copolymerization of three or more monomers is also performed, but copolymerization using two monomers M1 and M2 is discussed below for the sake of simplicity of the discussion. In addition, while copolymerization also takes place in ionic polymerizations, this database deals with only copolymerization in radical polymerization. [1,2,3]
Principle
Hereafter, it is assumed that the reactivity of the propagating radicals depends solely on the identity of the monomer unit at the propagating end and not on the chain length and chain compositions (the first-order Markov or terminal model). Then, two propagating radicals M1* and M2* formed from M1 and M2, respectively, react either M1 or M2 with the rate constant k11, k12, k21, and k22 giving M1* and M2*(Eqs 1 and 2). The k11 and k22 are the propagation constants of the homopolymerization (self-propagation) of M1 and M2, respectively, and the k12 and k21 are the cross-propagation constants between M1 and M2. The monomer feed at a certain monomer concentration, d[M1] / d[M2] ([M1] and [M2] are the molar concentration of M1 and M2, respectively) can be expressed as equation 3 with the parameters r1 and r2> termed as the monomer reactivity ratios. These parameters defined as (Eq 4) have been experimentally determined and are the subject of the current database and machine learning.
-
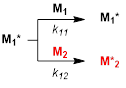 (1)
(1)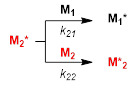 (2)
(2) -
d[M1] / d[M2] = { [M1] (r1 [M1] + [M2]) } / { [M2] ([M1] + r2 [M2]) }(3)
-
r1 = k11 / k12 and r2 = k22 / k21(4)
-
f1 = 1- f2 = [M1] / ([M1] + [M2])(5)
-
F1 = 1- F2 = d[M1] / (d[M1] + d[M2])(6)
-
F1 = (r1f12 + f1f2) / (r1f12 + 2f1f2 + r2 f22)(7)
Structure of the Copolymers
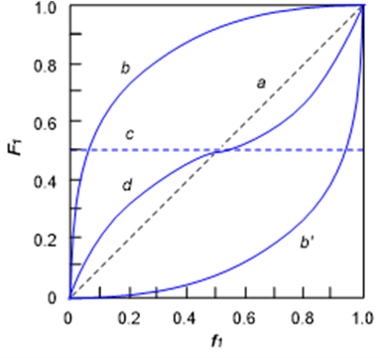
The copolymerization is termed ideal when r1r2 = 1 . The relative rates of incorporation of M1 monomer (and M2 monomer as well) into the copolymer are independent of the identity of M1* and M2*. The two subclasses of ideal copolymerization are described below.
1-1) r1 = r2 = 1 (Figure 1, a)
The probability of the propagating radicals M1* and M2* undergoing homo- and cross propagation reaction is the same. Therefore, F1 and f1 always have the same composition and show a linear relationship (Figure 1, a). The F1 in ideal copolymerization is the same as that in alternating copolymerization discussed below, but the microstructure of the former has a distribution of monomer sequences derived from statistical properties as schematically shown below.
1-2) r1 = 1/r2, r1 ≠ 1 (Figure 1, b and b’)
The F1/f1 plot is either convex or concave, depending on whether r1 is greater or less than unity (line b or c).
The propagating radicals M1* and M2* selectively react with M2 and M1, respectively, giving copolymers having alternating M1 and M2 sequences shown below. Thus, the F1 in the copolymer is always 0.5 regardless of the f1. Even both r1 and r2 are not 0, highly alternating copolymers are formed when both r1 and r2 are very small, close to 0.
Block copolymers with perfect controlled structure as shown below are commonly synthesized by living polymerization with sequential monomer addition technique. Therefore, they are not usually obtained in copolymerization in which multiple monomers are polymerized simultaneously. However, when both r1 and r2 are greater than unity, copolymers consisting of block-like sequences are formed.
When r1 and r2 parameters do not fall into one of the above categories, the copolymerization behaves differently. However, the behavior can be easily estimated from the above examples in either case.
4-1) r1, r2 < 1, r1, r2 ≠ 0
The propagating radicals M1* and M2* are more likely to react with the other monomer. Thus, the polymer composition curve takes an S-shape or inverse S-shape depending on the magnitude of r1 and r2. The plot has one intersection or crossover point with a line representing F1 = f1. The copolymerization at this point is termed azeotropic copolymerization because the composition of the copolymer does not change with the conversion of monomers.
When r1 = r2, the copolymer is richer in M1 than the feed mixture for f1 < 0.5 , and richer in M2 than the feed mixture for f1 > 0.5 with a crossover point at F1 = f1 = 0.5 (Figure 1, d). When r1 > r2 , the polymer composition curve becomes an unsymmetrical inverse S-shape with the crossover point shifting to F1 = f1 < 0.5 . When r1 < r2 , the opposite happens.
When r1 ≠ r2 , as the composition of monomer, f1, changes upon the progress of copolymerization, that of the copolymer, F1, also changes beside azeotropic copolymerization. This fact, combined with the presence of the termination reaction in the conventional radical polymerization, indicates that the finally obtained copolymers are a mixture of M1 rich and M2 rich copolymers depending on when the propagation occurs. In contrast, the same copolymerization is carried out under the living (reversible deactivation) conditions, gradient copolymers as schematically shown blow forms because no termination occurs.
4-2) r1 = 0, r2 < 1
The propagating radicals M2* add to M1 preferentially but M1 does not cross-propagate to M2. Therefore, the polymer composition, F1, can be close to 50% but does not exceed 50%. When r1 < 1, r2 = 0 , the opposite happens.
4-3) r1= 1, r2 < 1
The propagating radicals M2* add to M1 preferentially but M1 does not have selectivity, resulting in the formation of copolymer always rich in M1 fraction. No azeotropic copolymerization condition is found. When r1 < 1, r2 = 1 , the opposite happens.
4-4) r1 > 1, r2 < 1
Both propagating radicals M1 and M2* preferentially add to M1 monomer. Therefore, copolymers with M1 rich fraction always form as in 4-3) and 1-2). The shape of F1/f1 plot changes from sigmoidal to convex as the difference between r1 and r2 increases. When r1 < 1, r2 > 1 , the opposite happens.
References
- Odian G. Principles of Polymerization, Fourth Edition; John Wiley & Sons, Inc., Hoboken, New Jersey (2004).
- Hiemenz, P. C.; Lodge, T. P. Polymerr Chemistry, Second Edition; CRC Press, Boca Raton, FL (2007).
- Kamigaito, M.; Sato, K.; Sawamoto, M. In Polymer Synthesis; Endo, T. Ed. Kodansha Scientific, Tokyo (2010). [Japanese]